chillimath.com

chillimath

Menu

12. Find the 20th term of the series 2×4 + 4×6 + 6×8 + … + n terms.

Sequences and Series

Miscellaneous Exercise

Ncert Solutions Class 11 Chapter 8 Sequences and Series Miscellaneous Exercise Question 12
Previous
Next

Class 11

Sequences and Series

Miscellaneous Exercise

1. If f is a function satisfying f(x+y)=f(x)f(y) for all x,y∈N such that f(1)=3 and \(\sum_{x=1}^n f(x)=120\), find the value of n.

2. The sum of some terms of G.P. is 315 whose first term and the common ratio are 5 and 2, respectively. Find the last term and the number of terms.

3. The first term of a G.P. is 1. The sum of the third term and fifth term is 90. Find the common ratio of G.P.

4. The sum of three numbers in G.P. is 56. If we subtract 1,7,21 from these numbers in that order, we obtain an arithmetic progression. Find the numbers.

5. A G.P. consists of an even number of terms. If the sum of all the terms is 5 times the sum of terms occupying odd places, then find its common ratio.

6. If \(\frac{a+bx}{a-bx}=\)\(\frac{b+cx}{b-cx}=\)\(\frac{c+dx}{c-dx}\) \(x≠0\), then show that a,b,c and d are in G.P.

7. Let S be the sum, P the product and R the sum of reciprocals of n terms in a G.P. Prove that \(P^2 R^n=S^n\).

8. If a,b,c,d are in G.P, prove that \((a^n+b^n )\),\((b^n+c^n )\),\((c^n+d^n)\) are in G.P.

9. If a and b are the roots of \(x^2–3x+p=0\) and c, d are roots of \(x^2–12x+q=0\), where a,b,c,d form a G.P. Prove that (q+p) : (q–p) = 17:15.

10. The ratio of the A.M. and G.M. of two positive numbers a and b is m : n. Show that \(a:b=(m+\sqrt{m^2-n^2}):(m-\sqrt{m^2-n^2 })\).

11. Find the sum of the following series up to n terms:
(i) 5+55+555+ …….…
(ii) .6+.66+.666+…………..

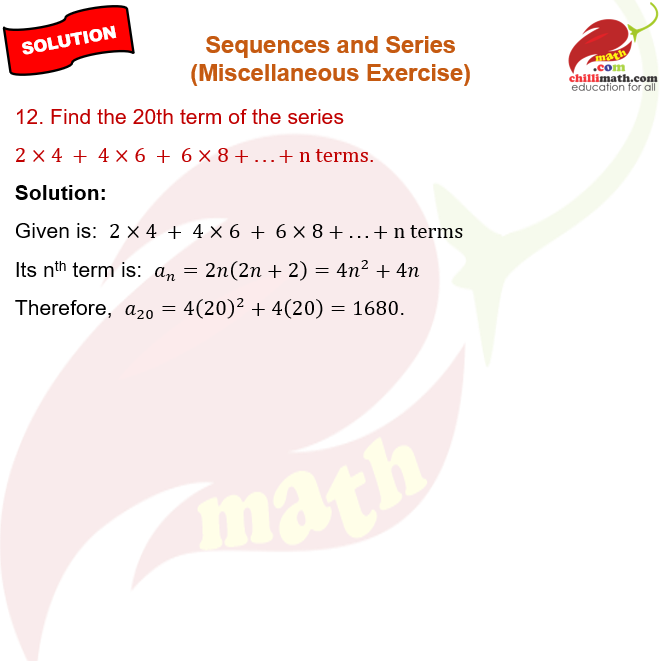
12. Find the 20th term of the series 2×4 + 4×6 + 6×8 + … + n terms.

13. A farmer buys a used tractor for Rs 12000. He pays Rs 6000 cash and agrees to pay the balance in annual installments of Rs 500 plus 12% interest on the unpaid amount. How much will the tractor cost him?

14. Shamshad Ali buys a scooter for Rs 22000. He pays Rs 4000 cash and agrees to pay the balance in annual installment of Rs 1000 plus 10% interest on the unpaid amount. How much will the scooter cost him?

15. A person writes a letter to four of his friends. He asks each one of them to copy the letter and mail to four different persons with instruction that they move the chain similarly. Assuming that the chain is not broken and that it costs 50 paise to mail one letter. Find the amount spent on the postage when 8th set of letter is mailed.

16. A man deposited Rs 10000 in a bank at the rate of 5% simple interest annually. Find the amount in 15th year since he deposited the amount and also calculate the total amount after 20 years.

17. A manufacturer reckons that the value of a machine, which costs him Rs. 15625, will depreciate each year by 20%. Find the estimated value at the end of 5 years.

18. 150 workers were engaged to finish a job in a certain number of days. 4 workers dropped out on second day, 4 more workers dropped out on third day and so on. It took 8 more days to finish the work. Find the number of days in which the work was completed.