chillimath.com

chillimath

Menu

13. Find \(\frac{dy}{dx}\), If \(y={sin}^{-1}{x}+{sin}^{-1}{\sqrt{1-x^2}}\),\(-1\le\ x\le1\).

Continuity and Differentiability

Miscellaneous Exercise

ncert solutions class 12 chapter 5 continuity and differentiability miscellaneous exercise question 13
Previous
Next

Class 12

Continuity and Differentiability

Miscellaneous Exercise

Differentiate w.r.t. x the function in Exercises 1 to 11.

1. \(\left(3x^2-9x+5\right)^9\).

2. \({sin}^3{x}+{cos}^6{x}\).

3. \(\left(5x\right)^{3cos{2}x}\).

4. \({sin}^{-1}{\left(x\sqrt x\right)}\),\(0\le\ x\le1\).

5. \(\frac{{cos}^{-1}{\frac{x}{2}}}{\sqrt{2x+7}}\),\(-2<x<2\).

6. \({cot}^{-1}{\left[\frac{\sqrt{1+sin{x}}+\sqrt{1-sin{x}}}{\sqrt{1+sin{x}}-\sqrt{1-sin{x}}}\right]}\),\(0<x<\frac{\pi}{2}\).

7. \(\left(log{x}\right)^{log{x}}\), \(x>1\).

8. \(cos{\left(acos{x}+bsin{x}\right)}\), for some constant a and b.

9. \(\left(sin{x}-cos{x}\right)^{\left(sin{x}-cos{x}\right)}\), \(\frac{\pi}{4}<x<\frac{3\pi}{4}\).

10. \(x^x+x^a+a^x+a^a\), for some fixed a>0 and x>0.

11. \(x^{x^2-3}+\left(x-3\right)^{x^2}\), for x>3.

12. Find \(\frac{dy}{dx}\), if \(y=12\left(1-cos{t}\right)\),\(x=10\left(t-sin{t}\right)\),\(-\frac{\pi}{2}<t<\frac{\pi}{2}\).

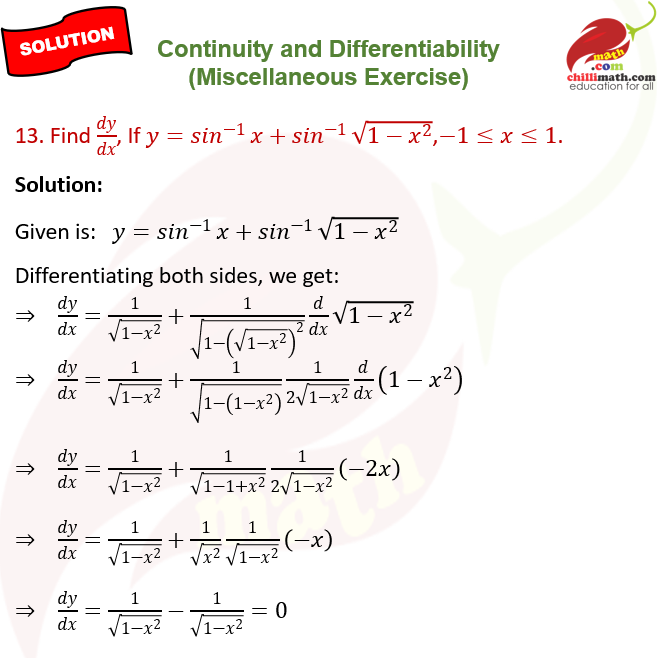
13. Find \(\frac{dy}{dx}\), If \(y={sin}^{-1}{x}+{sin}^{-1}{\sqrt{1-x^2}}\),\(-1\le\ x\le1\).

14. If \(x\sqrt{1+y}+y\sqrt{1+x}=0\), for \(-1<x<1\), prove that \(\frac{dy}{dx}=-\frac{1}{\left(1+x\right)^2}\).

15. If \(\left(x-a\right)^2+\left(y-b\right)^2=c^2\), for some c>0, prove that \(\frac{\left[1+\left(\frac{dy}{dx}\right)^2\right]^\frac{3}{2}}{\frac{d^2y}{dx^2}}\) is a constant independent of a and b.

16. If \(cos{y}=xcos{\left(a+y\right)} \) with \(cos{a}\neq\pm1\), prove that \(\frac{dy}{dx}=\frac{{cos}^2{\left(a+y\right)}}{sin{a}}\).

17. If \(x=a\left(cos{t}+tsin{t}\right) \) and \(y=a\left(sin{t}-tcos{t}\right)\), find \(\frac{d^2y}{dx^2}\).

18. If \(f\left(x\right)=\left|x\right|^3\), show that f”(x) exists for all real x and find it.

19. Using the fact that \(\sin{(A+B)}=\sin{A}\cos{B}+\cos{A}\sin{B}\) and the differentiation, obtain the sum formula for cosines.

20. Does there exist a function which is continuous everywhere but not differentiable at exactly two points? Justify your answer.

21. If \(y=\left|\begin{matrix}f\left(x\right)&g\left(x\right)&h\left(x\right)\\l&m&n\\a&b&c\\\end{matrix}\right|\), prove that \(\frac{dy}{dx}=\left|\begin{matrix}f'(x)&g'(x)&h'(x)\\l&m&n\\a&b&c\\\end{matrix}\right|\).

22. If \(y=e^{a{cos}^{-1}{x}}\), \(-1\le\ x\le1\), show that \(\left(1-x^2\right)\frac{d^2y}{dx^2}-x\frac{dy}{dx}-a^2y=0\).