chillimath.com

chillimath

Menu

Ncert Solutions for Class 9

Number Systems

Number Systems

Exercise 1.1

1. Is zero a rational number? Can you write it in the form \(\frac{p}{q}\), where p and q are integers and q ≠ 0?

2. Find six rational numbers between 3 and 4.

3. Find five rational numbers between \(3\over 5\) and \(4\over 5\).

4. State whether the following statements are true or false. Give reasons for your answers.

(i) Every natural number is a whole number.

(ii) Every integer is a whole number.

(iii) Every rational number is a whole number.

Number Systems

Exercise 1.2

1. State whether the following statements are true or false. Justify your answers.

(i) Every irrational number is a real number.

(ii) Every point on the number line is of the form \(\sqrt{m}\), where m is a natural number.

(iii) Every real number is an irrational number.

2. Are the square roots of all positive integers irrational? If not, give an example of the square root of a number that is a rational number.

3. Show how \(\sqrt5\) can be represented on the number line.

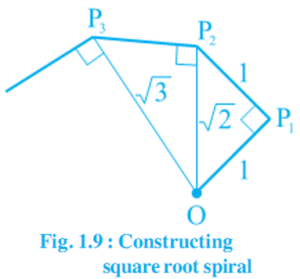
4. Classroom activity (Constructing the ‘square root spiral’) :  Take a large sheet of paper and construct the square root spiral in the following fashion. Start with a point O and draw a line segment OP1 of unit length. Draw a line segment P1P2 perpendicular to OP1 of unit length (see Fig. 1.9). Now draw a line segment P2P3 perpendicular to OP2. Then draw a line segment P3P4 perpendicular to OP3. Continuing in this manner, you can get the line segment Pn-1Pn by drawing a line segment of unit length     perpendicular to OPn-1. In this manner, you will have created the points P2, P3,…., Pn, … ., and joined them to create a beautiful spiral depicting \(\sqrt 2\), \(\sqrt 3\), \(\sqrt 4\), …

Number Systems

Exercise 1.3

1. Write the following in decimal form and say what kind of decimal expansion each has:

(i) \(36\over 100\)   

(ii) \(1\over 11\)                     

(iii) \(4{1\over 8}\)                   

(iv) \(3\over 13\)

(v) \(2\over 11\)                      

(vi) \(329\over 400\)

2. You know that \({1\over 7}=0.\overline{142857}\). Can you predict what the decimal expansion of \(2\over 7\), \(3\over 7\), \(4\over 7\), \(5\over 7\), \(6\over 7\) are without actually doing the long division? If so, how?

3. Express the following in the form \(p\over q\), where p and q are integers and q ≠ 0.

(i) \(0.\overline{6}\)

(ii) \(0.4\overline{7}\)

(iii) \(0.\overline{001}\)

4. Express 0.99999 …. in the form \(p\over q\). Are you surprised by your answer? With your teacher and classmates discuss why the answer makes sense.

5. What can the maximum number of digits be in the repeating block of digits in the decimal expansion of \(1\over 17\)? Perform the division to check your answer.

6. Look at several examples of rational numbers in the form \(p\over q\) (q ≠ 0), where p and q are integers with no common factors other than 1 and having terminating decimal representations (expansions). Can you guess what property q must satisfy?

7. Write three numbers whose decimal expansions are non-terminating non-recurring.

8. Find three different irrational numbers between the rational numbers \(5\over 7\) and \(9\over 11\).

9. Classify the following numbers as rational or irrational:

(i) \(\sqrt{23}\)

(ii) \(\sqrt{225}\)

(iii) 0.3796

(iv) 7.478478…             

(v) 1.101001000100001…

Number Systems

Exercise 1.4

1. Classify the following numbers as rational or irrational:

(i) \(2-\sqrt 5\)

(ii) \((3+\sqrt{23})-\sqrt{23}\)

(iii) \({2\sqrt 7}\over{7\sqrt 7}\)

(iv) \(1\over {\sqrt 2}\)

(v) \(2\pi\)

2. Simplify each of the following expressions:

(i) \((3+\sqrt 3)(2+\sqrt 2)\)

(ii) \((3+\sqrt 3)(3-\sqrt 3)\)

(iii) \((\sqrt 5+\sqrt 2)^2\)     

(iv) \((\sqrt 5-\sqrt 2)(\sqrt 5+\sqrt 2)\)

3. Recall, π is defined as the ratio of the circumference (say c) of a circle to its diameter (say d). That is, \(\pi={c\over d}\)·  This seems to contradict the fact that π is irrational. How will you resolve this contradiction?

4. Represent \(\sqrt {9.3}\) on the number line.

5. Rationalise the denominators of the following:

(i) \(1\over{\sqrt 7}\)

(ii) \(1\over{\sqrt 7-\sqrt 6}\)  

(iii) \(1\over{\sqrt 5+\sqrt 2}\)

(iv) \(1\over{\sqrt 7-2}\)

Number Systems

Exercise 1.5

1. Find:

(i) \(64^{1\over 2}\)

(ii) \(32^{1\over 5}\)                     

(iii) \(125^{1\over 3}\)

2. Find:

(i) \(9^{3\over 2}\)

(ii) \(32^{2\over 5}\)

(iii) \(16^{3\over 4}\)   

(iv) \(125^{-1\over 3}\)

3. Find:

(i) \({2^{2\over 3}}{2^{1\over 5}}\)

(ii) \(({1\over {3^3}})^7\)  

(iii) \({11^{1\over 2}}\over{11^{1\over 4}}\) 

(iv) \({7^{1\over 2}}{8^{1\over 2}}\)