Class 12
Inverse Trigonometric Functions
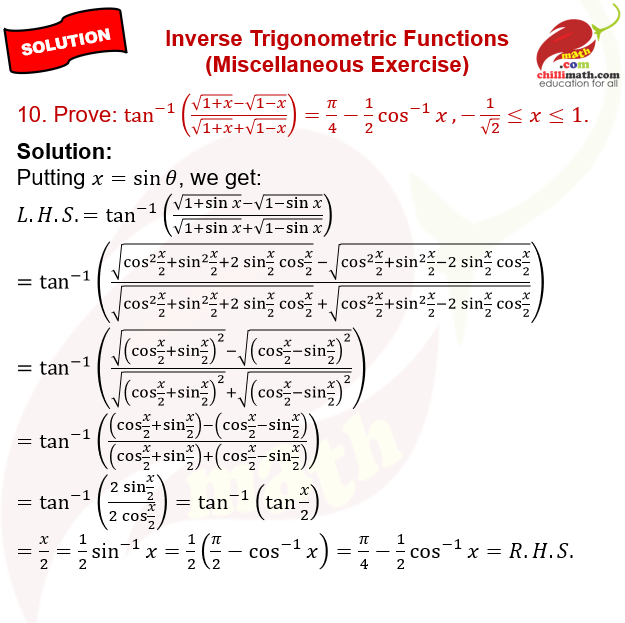
Miscellaneous Exercise
Find the value of the following:
1. \(cos^{-1}(cos\frac{13\pi}{6})\).
2. \(tan^{-1}(tan\frac{7\pi}{6})\).
3. \(2sin^{-1}{\frac{3}{5}}\)\(=tan^{-1}{\frac{24}{7}}\).
4. \(sin^{-1}{\frac{8}{17}}+sin^{-1}{\frac{3}{5}}\)\(=tan^{-1}{\frac{77}{36}}\).
5. \(cos^{-1}{\frac{4}{5}}+cos^{-1}{\frac{12}{13}}\)\(=cos^{-1}{\frac{33}{65}}\).
6. \(cos^{-1}{\frac{12}{13}}+sin^{-1}{\frac{3}{5}}\)\(=sin^{-1}{\frac{56}{65}}\).
7. \(tan^{-1}{\frac{63}{16}}=sin^{-1}{\frac{5}{13}}\)\(+cos^{-1}{\frac{3}{5}}\).
8. \(tan^{-1}{\sqrt{x}}=\frac{1}{2}cos^{-1}{\frac{1-x}{1+x}}\).
Solve the following equations:
11. \(2tan^{-1}(cosx)=tan^{-1}(2cosecx)\).
12. \(tan^{-1}{\frac{1-x}{1+x}}\)\(=\frac{1}{2}tan^{-1}x,\;(x>0)\)
13. \(sin(tan^{-1}x),|x|<1\) is equal to:
(A) \(\frac{x}{\sqrt{1-x^2}}\)
(B) \(\frac{1}{\sqrt{1-x^2}}\)
(C) \(\frac{1}{\sqrt{1+x^2}}\)
(D) \(\frac{x}{\sqrt{1+x^2}}\)
14. \(sin^{-1}(1-x)-2sin^{-1}x\)\(=\frac{\pi}{2}\), then x is equal to: