chillimath.com

chillimath

Menu

13. Show that the relation R defined in the set A of all polygons as R={(P1,P2) : P1 and P2 have same number of sides}, is an equivalence relation. What is the set of all elements in A related to the right angle triangle T with sides 3,4 and 5?

Relations and Functions

Exercise 1.1

Ncert solutions class 12 chapter 1 exercise 1
Ncert solutions class 12 chapter 1 exercise 1
Previous
Next

Class 12

Relations and Functions

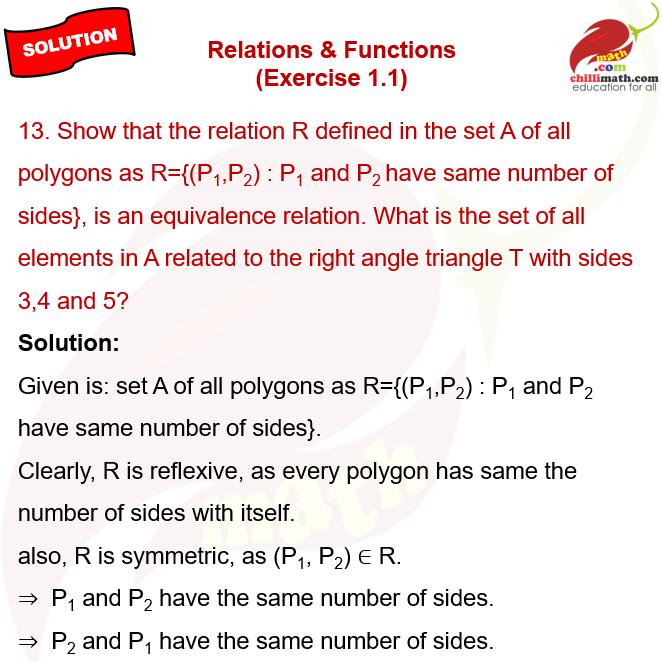
Exercise 1.1

1. Determine whether each of the following relations are reflexive, symmetric and transitive:

(i) Relation R in the set A={1,2,3,…,13,14} defined as R={(x,y) : 3x–y=0}

(ii) Relation R in the set N of natural numbers defined as R={(x,y) : y=x+5 and x<4}

(iii) Relation R in the set A={1,2,3,4,5,6} as R={(x,y) : y is divisible by x} 

(iv) Relation R in the set Z of all integers defined as R={(x,y) : x–y is an integer}

(v) Relation R in the set A of human beings in a town at a particular time given by:

(a)  R={(x, y) : x and y work at the same place}

(b)  R={(x, y) : x and y live in the same locality}

(c)  R={(x, y) : x is exactly 7 cm taller than y}

(d)  R={(x, y) : x is wife of y}

(e)  R={(x, y) : x is father of  y}

2. Show that the relation R in the set R of real numbers, defined as R={(a,b) : a≤b2} is neither reflexive nor symmetric nor transitive.

3. Check whether the relation R defined in the set {1,2,3,4,5,6} as R={(a,b) : b=a+1} is reflexive, symmetric or transitive.

4. Show that the relation R in R defined as R={(a,b) : a≤b}, is reflexive and transitive but not symmetric.

5. Check whether the relation R in R defined by R={(a,b) : a≤b3} is reflexive, symmetric or transitive.

6. Show that the relation R in the set {1,2,3} given by R={(1,2), (2,1)} is symmetric but neither reflexive nor transitive.

7. Show that the relation R in the set A of all the books in a library of a college, given by R={(x,y) : x and y have same number of pages} is an equivalence relation.

8. Show that the relation R in the set A={1,2,3,4,5} given by R={(a,b) : |a–b| is even}, is an equivalence relation. Show that all the elements of {1,3,5} are related to each other and all the elements of {2,4} are related to each other. But no element of {1,3,5} is related to any element of {2,4}.

9. Show that each of the relation R in the set A={x\(\in\)Z : 0≤x≤12}, given by

(i) R={(a,b) : |a–b| is a multiple of 4}

(ii) R={(a,b) : a=b} 

is an equivalence relation. Find the set of all elements related to 1 in each case.

10. Give an example of a relation. Which is:

(i) Symmetric but neither reflexive nor transitive.

(ii) Transitive but neither reflexive nor symmetric.

(iii) Reflexive and symmetric but not transitive.

(iv) Reflexive and transitive but not symmetric.

(v) Symmetric and transitive but not reflexive.

11. Show that the relation R in the set A of points in a plane given by R={(P,Q) : distance of the point P from the origin is same as the distance of the point Q from the origin}, is an equivalence relation. Further, show that the set of all points related to a point P≠(0,0) is the circle passing through P with origin as centre.

12. Show that the relation R defined in the set A of all triangles as R={(T1,T2) : T1 is similar to T2}, is equivalence relation. Consider three right angle triangles T1 with sides 3,4,5, T2 with sides 5,12,13 and T3 with sides 6,8,10. Which triangles among T1,T2 and T3 are related?

13. Show that the relation R defined in the set A of all polygons as R={(P1,P2) : P1 and P2 have same number of sides}, is an equivalence relation. What is the set of all elements in A related to the right angle triangle T with sides 3,4 and 5?

14. Let L be the set of all lines in XY plane and R be the relation in L defined as R={(L1,L2) : L1 is parallel to L2}. Show that R is an equivalence relation. Find the set of all lines related to the line y=2x+4.

15. Let R be the relation in the set {1,2,3,4} given by R={(1,2), (2,2), (1,1), (4,4), (1,3), (3,3), (3,2)}. Choose the correct answer.

(A) R is reflexive and symmetric but not transitive.

(B) R is reflexive and transitive but not symmetric.

(C) R is symmetric and transitive but not reflexive.

(D) R is an equivalence relation.

16. Let R be the relation in the set N given by R={(a,b) : a=b–2, b>6}. Choose the correct answer.

(A) (2,4) \(\in\) R      

(B) (3,8) \(\in\) R 

(C) (6,8) \(\in\) R 

(D) (8,7) \(\in\) R