Ncert Solutions for Class 10 Introduction to Trigonometry

Ncert Solutions for Class 10

Introduction to Trigonometry

• Exercise 8.1

• Exercise 8.2

Introduction to Trigonometry

Exercise 8.1

1. In ΔABC, right-angled at B, AB = 24 cm, BC = 7 cm. Determine:
(i) sin A, cos A 
(ii) sin C, cos C

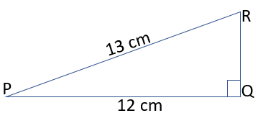
2. In Figure, find tan P – cot R.

3. If sin A = (frac{3}{4}), calculate cos A and tan A.

4. Given 15 cot A = 8, find sin A and sec A.

5. Given sec θ = (frac{13}{12}), calculate all other trigonometric ratios.

6. If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B.

7. If cot θ = (frac{7}{8}), evaluate:
(i) (frac{(1+sin⁡θ)(1-sin⁡θ)}{(1+cos⁡θ)(1-cos⁡θ)})

(ii) (cot^2⁡θ)

8. If 3 cot A = 4, check whether (frac{1-tan^2⁡A}{1+tan^2⁡A}=cos^2 A-sin^2 A ) or not.

9. In triangle ABC, right- angled at B, if tan A = (frac{1}{sqrt{3}}), find the value of:
(i) sin A cos C + cos A sin C
(ii) cos A cos C – sin A sin C

10. In ΔPQR, right-angled at Q, PR + QR = 25 cm and PQ = 5 cm. Determine the values of sinP, cosP and tanP.

11. State whether the following are true or false. Justify your answer.
(i) The value of tan A is always less than 1.
(ii) sec A = (frac{12}{5}) for some value of angle A.
(iii) cos A is the abbreviation used for the cosecant of angle A.
(iv) cot A is the product of cot and A.
(v) sin θ = (frac{4}{3}) for some angle θ.