chillimath.com

chillimath

Menu

4. Find the intervals in which the function f given by \(f\left(x\right)=x^3+\frac{1}{x^3},x\neq0\) is
(i) increasing
(ii) decreasing.

Application of Derivatives

Miscellaneous Exercise

Ncert Solutions Class 12 chapter 6 Application of Derivatives Miscellaneous Exercise Question 4
Previous
Next

Class 12

Application of Derivatives

Miscellaneous Exercise

1. Show that the function given by \(f\left(x\right)=\frac{log{x}}{x}\) has maximum at x=e.

2. The two equal sides of an isosceles triangle with fixed base b are decreasing at the rate of 3 cm per second. How fast is the area decreasing when the two equal sides are equal to the base ?

3. Find the intervals in which the function f given by \(f\left(x\right)=\frac{4sin{x}-2x-xcos{x}}{2+cos{x}}\) is
(i) increasing
(ii) decreasing.

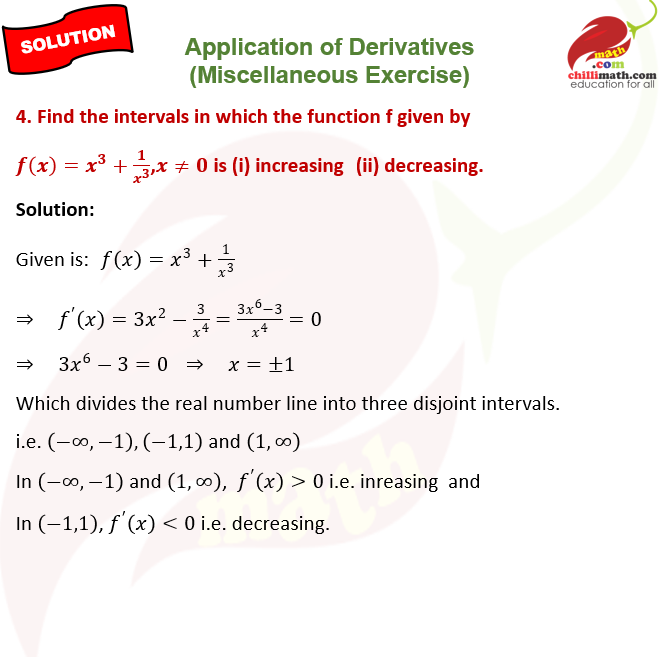
4. Find the intervals in which the function f given by \(f\left(x\right)=x^3+\frac{1}{x^3},x\neq0\) is
(i) increasing
(ii) decreasing.

5. Find the maximum area of an isosceles triangle inscribed in the ellipse \(\frac{x^2}{a^2}+\frac{y^2}{b^2}=1\) with its vertex at one end of the major axis.

6. A tank with rectangular base and rectangular sides, open at the top is to be constructed so that its depth is 2 m and volume is 8 m3. If building of tank costs Rs 70 per sq metres for the base and Rs 45 per square metre for sides. What is the cost of least expensive tank?

7. The sum of the perimeter of a circle and square is k, where k is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle.

8. A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening.

9. A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle. Show that the maximum length of the hypotenuse is \((a^{2/3}+b^{2/3}\ )^{3/2}\).

10. Find the points at which the function f given by \(f(x)=(x–2)^4 (x+1)^3\) has
(i) local maxima
(ii) local minima
(iii) point of inflexion

11. Find the absolute maximum and minimum values of the function f given by \(f(x)=\cos^2{x}+\sin{x},\ x\in[0,\pi]\).

12. Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is \(\frac{4r}{3}\).

13. Let f be a function defined on [a,b] such that f’(x)>0, for all \(x\in(a,b)\). Then prove that f is an increasing function on (a,b).

14. Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is \(\frac{2R}{\sqrt3}\). Also find the maximum volume.

15. Show that height of the cylinder of greatest volume which can be inscribed in a right circular cone of height h and semi vertical angle α is one-third that of the cone and the greatest volume of cylinder is \(\frac{4}{27}\pi\ h^3{tan}^2{\alpha}\).

16. A cylindrical tank of radius 10 m is being filled with wheat at the rate of 314 cubic metre per hour. Then the depth of the wheat is increasing at the rate of
(A) \(1  \ m^3/h\)
(B) \(0.1 \  m^3/h\)
(C) \(1.1   \ m^3/h\)
(D) \(0.5  \ m^3/h\)